Program for computing seismic hazard

Attenuation Table Montalva et al., 2017

  • Attenuation Table Montalva et al., 2017

Attenuation Table Montalva et al., 2017

  • 218 Views
  • Last Post 11 December 2019
Marisella Ortega posted this 02 December 2019

Hello, I am writing because I am doing a study of seismic hazard in Chile and I need to test the attenuation model for Chilean earthquakes of Montalva et al., 2017. You have entered it in R-CRISIS, but when I try it I don't have values for PGA, the spectral values start from 0.01s and in addition the results are quite high, close to 1.5[g]. I imagine it could be because of the standard deviation that is quite large in the model that comes loaded. Therefore, I would like to ask you if you can send me the excel file that contains the equation of Montalva et al., 2017 and with which the .atn file is created to upload it to R-CRISIS and modify it as I want. Is it possible? This would be of great help to me and this project.

 

Thank you very much,

Marisella Ortega.

Order By: Standard | Newest | Votes
Content_Manager posted this 09 December 2019

Dear Marisella,

All GMPMs available in R-CRISIS, prior to add, are previously revisited and validated against the results provided by authors in their papers. In some cases, authors provide Excel spreadsheets or Matlab scripts in order to make the verification more easily. 

Regarding Montalva et al. (2017) GMPM, the validation was made graphically; you may found it Chapter 4, section 4.5.2 Graphical comparisons, available in R-CRISIS Documentation. You can be sure that the model is correctly built in R-CRISIS.

 

Thank you for preference and interest in R-CRISIS.

 

Best regards,

Marisella Ortega posted this 10 December 2019

Ok. So, to prove it the right way, could you tell me what the values of "Sigma truncation" and "Units coefficient" correspond to? I imagine that for rock it is good to put a Vs30 of 1000. I attach a picture of what I should enter.

Thank you very much,

Marisella Ortega.

Content_Manager posted this 11 December 2019

Dear Marisella,

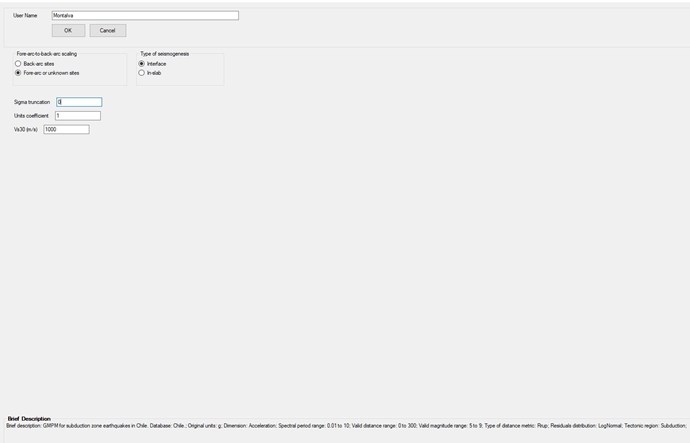
The detailed explanation of "Sigma truncation" is in the R-CRISIS Help Menu -> Building a data file -> 10-Attenuation Data. In this section, you will find a link to "Probabilistic interpretation of attenuation relations".

On the other hand, here is the explanation for "Units coefficients" (text extracted from R-CRISIS Help Menu):

All GMPM used by R-CRISIS (attenuation tables, built-in models and generalized models) give probabilistic relations between earthquake properties, site characteristics and intensities at a site. These relations are given in terms of the first and second statistical moments of the intensities, given some earthquake and site parameters. For instance, for the GMPM in which the residuals are lognormally distributed, the GMPM gives the median and the standard deviation of the natural logarithm of the intensity, given a set of earthquake and site parameters.

The GMPM are constructed to express the intensities in certain units. These units are called the "original units". However, the user of CRISIS might want to perform calculations for intensities expressed in other units. For instance, a user could be using a model whose original units are cm/sec2, but he wants to make hazard calculations for intensities expressed as a fraction of the acceleration of gravity, g. In these case, the g units will be called the "user units", which are given by the user in the Intensities Screen.

The Units Coefficient (UC) is a positive number used to change from the model's original units to the user units, using the following relation:

  1 user unit = UC original units (1)

For instance, in the example given above, since the original units are cm/sec2 and the user units are fractions of g, we would have that 1 g = UC cm/sec2, and hence UC=981, because 1 g = 981 cm/sec2.

Regarding the Vs30 value, Montalva et al. (2017) consider Rock condition as Vs30=1000 m/s, you may check this condition in their work.

 

Do not hesitate to contact us if you have further questions.

 

Thank you for your preference and interest in R-CRISIS.

 

Best regards,

 

Do you want to make a question?

Please sign in
Categories
Search
Popular Tags
Hot Topics
Close